The systematic study of spherical tilings goes back to 1924 ([2], p.467) but

their combinatorial classification is yet an open problem. Our aim is the development of computational GeoGebra tools enhancing the research on spherical tilings.

Considering a particular class of tilings, namely some monohedral spherical tilings [1, 3], the testing with some of the developed tools are very encouraging, proving to be quite useful instruments (see figure 1) comparing with the classical methods, where computational means have been essentially used for visualization. The potential of the tools developed allow an interactive way of guiding the study process, combining the possibility of changing, among others, the initial conditions to the possibility of computational validation of conjectures, besides the immediate visualization of the tilling studied/found.

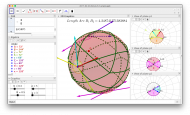
Figure 1: Image of application of GeoGebra used to undercover the tiling B2/5.
.

In this presentation I will explain some of the results already achieved, as well as the tools developed for this purpose. The outline of the algorithms behind some of the new designed and implemented GeoGebra tools and applications will be given. Finally we point out some of the limitations of the work developed and present some ideas for future work.

References

[1] A. M. A. Breda. A class of tilings of s2. Geometriae Dedicata, 44(3):241–253, 1992.
[2] R. J. M. Dawson. Tilings of the sphere with isosceles triangles. Discrete & Computational Geometry, 30(3):467–487, 2003.
[3] Y. Ueno, Y. Agaoka, et al. Classification of tilings of the 2-dimensional sphere by congruent triangles. Hiroshima Mathematical Journal, 32(3):463–540, 2002.